Cauchy problem for dispersive equations

Sep 1, 1991

I have been interested in the local or global in time Cauchy problem in some Sobolev spaces for various dispersive equations in the class of non-linear Schrödinger equations coupled to matter equations. Some asymptotic limit equations have been investigated.

I also studied a general framework for the Poisson’s recurrence for Hamiltonian equations and their numerical time discretizations.

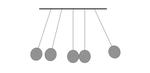
More recently I addressed the $p$-Schrödinger equation for the modelling of Newton’s cradle.

My PhD thesis deals with the theme, as well as part of my Habilitation thesis.

Other
Brigitte Bidegaray-Fesquet
Brigitte Bidegaray-Fesquet
Researcher

Publications

From Newton's cradle to the discrete p-Schrödinger equation
We investigate the dynamics of a chain of oscillators coupled by fully-nonlinear interaction potentials. This class of models includes …
Brigitte Bidegaray-Fesquet, Eric Dumas, Guillaume James
Cite Project DOI
On the propagation of an optical wave in a photorefractive medium
The aim of this paper is first to review the derivation of a model describing the propagation of an optical wave in a photorefractive …
Brigitte Bidegaray-Fesquet, Jean-Claude Saut
Cite Project DOI
A non local Schrödinger model for the propagation of waves in a photorefractive medium
We present various mathematical results (Cauchy problem, solitary waves) for the Zozulya–Anderson model which describes the …
Brigitte Bidegaray-Fesquet
Cite Project
Contributions à l'électromagnétisme dans le domaine temporel. Modélisation classique et quantique en optique non linéaire
Brigitte Bidégaray
Cite Project Project Project
The Cauchy Problem for Schrödinger-Debye equations
In this article we study the local-in-time Cauchy problem for the Schrödinger-Debye equations. This model occurs in nonlinear optics …
Brigitte Bidégaray
Cite Project DOI
On the Cauchy problem for some systems occurring in nonlinear optics
We study the Cauchy problem for two systems of equations (Maxwell-Debye and Maxwell-Bloch) describing laser-matter interaction …
Brigitte Bidégaray
Cite Project Project
A perturbative analysis of the time-envelope approximation in strong Langmuir turbulence
We investigate a nonlinear set of coupled-wave equations describing the inertial regime of the strong Langmuir turbulence, namely …
Luc Bergé, Brigitte Bidégaray, Thierry Colin
Cite Project DOI
On a nonlocal Zakharov equation
Brigitte Bidégaray
Cite Project DOI
Invariant measures for some partial differential equations
We construct invariant measures for Hamiltonian systems such as the nonlinear Schrrdinger equation or the wave equation in order to …
Brigitte Bidégaray
Cite Project DOI
Etude qualitative d'équations d'ondes dispersives
Cette thèse comporte trois parties traitant différents aspects de l’étude des équations d’ondes dispersives. Les modèles …
Brigitte Bidégaray
Cite Project
Etude d'équations intervenant en optique non linéaire
On étudie le problème de Cauchy associé à deux systèmes d’équations (Maxwell-Debye et Maxwell-Bloch) décrivant des phénomènes …
Brigitte Bidégaray
Cite Project Project
On a nonlocal Zakharov equation
This article deals with the Cauchy problem for a nonlocal Zakharov equation. We will first recall the physical motivation (due to V.E. …
Brigitte Bidégaray
Cite Project