Les déterminants de Sylvester

Pour Takakazu, comme pour Leibniz, Cramer ou Vandermonde, la résolution d'un système linéaire n'était qu'un cas particulier du problème général de l'élimination des variables entre des équations polynomiales. Il consiste à trouver des solutions communes à un système d'équations polynomiales à plusieurs variables. Le système est linéaire si les degrés des polynômes en chacune des variables est $1$. L'autre cas particulier facile est celui où il n'y a qu'une variable et deux équations polynomiales. Il s'agit donc de déterminer une racine commune à deux polynômes, disons

$$P=a_0+a_1X+\cdots+a_nX^n$$   et$$\quad Q=b_0+b_1X+\cdots+b_mX^m\;.$$

Quelle condition doivent satisfaire les coefficients pour que $P$ et $Q$ aient une racine commune ? Commençons par former la matrice de Sylvester, $S(P,Q)$, en écrivant $m+n$ colonnes obtenues en répétant de façon décalée les deux vecteurs de coefficients. Pour $n=2$ et $m=3$ :

$$S(P,Q) = \left(\begin{array}{ccccccc} a_0 &0 &0 &b_0 &0 &0 &0\\ ... ..._2 &b_1\\ 0 &0 &0 &0 &0 &b_3 &b_2\\ 0 &0 &0 &0 &0 &0 &b_3 \end{array}\right)$$

Théorème 4   Le degré du $\mathrm{pgcd}$ de $P$ et $Q$ et le rang de la matrice $S(P,Q)$ sont liés par :

$$\mathrm{deg} \mathrm{pgcd}(P,Q) = n+m-\mathrm{rang} S(P,Q)\;.$$

Vous pouvez démontrer vous-mêmes ce résultat : il suffit de comprendre quel est le rapport entre l'arithmétique des polynômes et le noyau de la matrice de Sylvester. Notons $R$ le $\mathrm{pgcd}$ de $P$ et $Q$ et supposons qu'il soit de degré supérieur ou égal à $1$. Notons $A$ et $B$ les quotients de $P$ et $Q$ par $R$ : ils sont tels que $P=AR$ et $Q=BR$, donc $BP-AQ=0$. Les polynômes $A$ et $B$ sont de degrés au plus $n-1$ et $m-1$. Écrivons :

$$A=\alpha_0+\alpha_1X+\cdots+\alpha_{n-1}X^{n-1}$$   et$$\quad B=\beta_0+\beta_1X+\cdots+\beta_{m-1}X^{m-1}\;.$$

Soit $v$ le vecteur de $\mathbb{R}^{n+m}$ obtenu en concaténant les coefficients de $B$ puis de $-A$.

$$v=(\beta_0,\beta_1,\ldots,\beta_{m-1}, -\alpha_0,-\alpha_1,\ldots,-\alpha_{n-1})\;.$$

Convainquez-vous que le produit de la matrice $S(P,G)$ par le vecteur colonne ${^t\!v}$ est le vecteur des coefficients de $BP-AQ$. Le reste devrait être facile. Le déterminant de la matrice de Sylvester s'appelait au début le résultant. Il était déjà implicitement présent chez Takakazu comme chez Leibniz, et avait été explicité par Euler, puis Bézout. Pourquoi donc lui a-t-on donné le nom de James Joseph Sylvester (1814-1897) ? Certainement pas à l'initiative de ses étudiants de l'Université de Virginie. Il était pourtant prévenu : en tant que juif, et anglais de surcroît, il n'allait pas être facilement accepté par les «White Anglo-Saxon Protestants » de l'état esclavagiste qu'était encore la Virginie d'avant la guerre de sécession (l'histoire se déroule début 1842). ⁶.

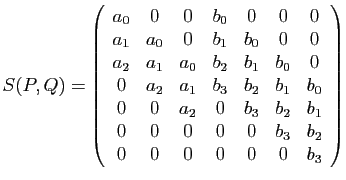
Unless Sylvester is content to sink into a mere cypher, and submit to all sorts of imposition from the students, he will have difficulties. They will try him, and when they do so, he will [...] commit some sort of blunder, and compromise his dignity in some way. I reckon our London cockney knows about as much about Virginian manners and character as a horse would about differential calculus.

Effectivement, la provocation des étudiants et la gaffe de Sylvester ne s'étaient pas faites attendre.

The cause of his sudden abandonment of the University of Virginia is often related by the Rev. R.L. Dabney, as follows: In Sylvester's class were a pair of brothers, stupid and excruciatingly pompous. When Sylvester pointed out the blunders made in a recitation by the younger of the pair, this individual felt his honor and family pride aggrieved, and sent word to Professor Sylvester that he must apologize or be chastised.

Sylvester bought a sword-cane, which he was carrying when way-laid by the brothers, the younger armed with a heavy bludgeon.

An intimate friend of Dr. Dabney's happened to be approaching at the moment of the encounter. The younger brother stepped up in front of Professor Sylvester and demanded an instant and humble apology.

Almost immediately he struck at Sylvester, knocking off his hat, and then delivered with his heavy bludgeon a crushing blow upon Sylvester's bare head.

Sylvester drew his sword-cane and lunged straight at him, striking him just over the heart. With a despairing howl, the student fell back into his brother's arms screaming out, ``I am killed!!'' ``He has killed me!'' Sylvester was urged away from the spot by Dr. Dabney's friend, and without even waiting to collect his books, he left for New York, and took ship back to England.

Meanwhile, a surgeon was summoned to the student, who was lividly pale, bathed in cold sweat, in complete collapse, seemingly dying, whispering his last prayers. The surgeon tore open his vest, cut open his shirt, and at once declared him not in the least injured. The fine point of the sword-cane had struck a rib fair, and caught against it, not penetrating.

When assured that the wound was not much more than a mosquito-bite, the dying man arose, adjusted his shirt, buttoned his vest, and walked off, though still trembling from the nervous shock.